Elastic 2D modelling including the free surface
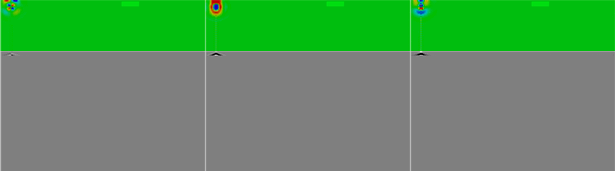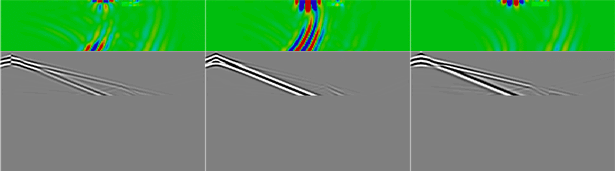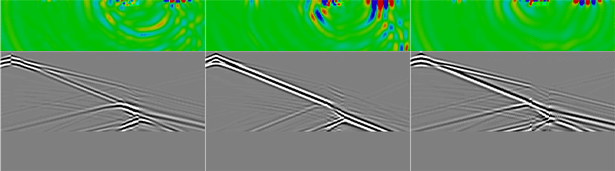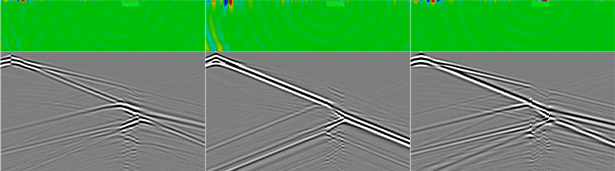
This is an example of a 2-dimensional numerical elastic simulation. The table shows the modelling parameters. The animation shows the seismogram section (bottom) and a series of snapshots (top) at times delta_t apart. The snapshots are displayed together with the step-like subsurface structure. The seismogram section grows with time according to the time of the displayed snapshot.
Here three different representations for the modelling results are given. From left to right horizontal and vertical particle velocity and pressure amplitudes are given.
Model parameters
| nx | 225 |
| nz | 81 |
| delta_x | 10 m |
| delta_z | 10 m |
| delta_t | 50 ms |
| t_max | 1.5 s |
| f_max | 50 Hz |
| vp_1 | 1500 m/s |
| vs_1 | 1000 m/s |
| rho_1 | 1500 kg/m^3 |
| vp_2 | 3000 m/s |
| vs_2 | 2000 m/s |
| rho_2 | 2000 kg/m^3 |
The source is a vertical point force at a depth of 10 gridpoints which generates P- and S-waves. At the surface Rayleigh-waves are generated. A box-like near (light green) surface inhomogeneity (higher velocities) produces diffracted body waves at its corners. It also patriallly reflects the surface wave which then travels in the opposite direction. The near surface inhomogeity partially traps the surface wave. This can also be seen in the seismograms.
Reference:
Kosloff, D., Kessler, D., Filho, A.Q., Tessmer, E., Behle, A., Strahilevitz, R., 1990,
Solution of the equations of dynamic elasticity by a Chebyshev spectral method,
Geophysics, 55, 734-748.